You're a teacher in a public school.  You give your 3rd graders a worksheet of 50 two digit addition questions, e.g. 43+25.  The kid gets 90% of them right. Pass or fail?

II.

Now it's reading comprehension time, you give the kid this:





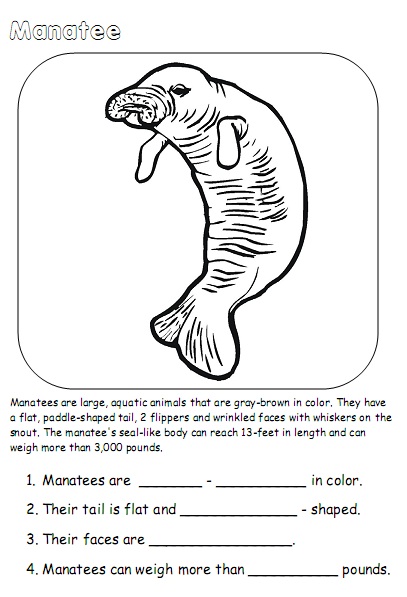
He gets them all right.  So?

III.


The math example, above, is real, and real(ly) scary.



     4 5
+   2 4
______
     6 9


So the kid had done an entire worksheet of these.  But what was hidden from view was that  the kid had absolutely no idea he was adding 2 digit numbers.  He had memorized the mechanics of the process and nothing else.  So when he was asked, "if you have 45 beads, and a friend gives you 24 beads, how many beads to you have?" he didn't know to put the numbers on top of each other like the worksheet; what he did was say the number 45 and then start counting on his fingers, 46, 47, 48, 49.... and of course he ran out of fingers and had no idea when to stop, so he guessed.

The problem is that as long as he completed the worksheet, you wouldn't know there was a problem with doing math until it was way too late.  If the kid is clever in other ways-- say, fast at finger counting-- he could easily convey the impression that he understands how to add 2 digit numbers, and what that means, and so everyone thinks he's progressing just fine; only to reach a later point when his clever shortcut is too primitive to work.  Now suddenly you have a 6th grader who appears to falling behind.  But he was never really caught up.   I suspect that this almost entirely explains Americans' universal hatred of word problems.

IV.


A second maddeningly infuriating example.  There's a summer class of 1st graders with behavioral problems who are learning to read.  Granted a unique sample set, but it's the only one I have. Ok, so one boy is reading the story of Aladdin.

I notice that he is reading the words but there's no cadence, there's no rhythm.  At times, he'll read the first words of a second sentence into the flow of the first sentence, i.e. "Aladdin took the lamp.  Jasmine polished it." becomes "Aladdin, took the lamp Jasmine polished.  It..."

However, he is reading the words correctly.  So?  So the only way you can evaluate his comprehension is to ask him questions, which he answers with little hesitation. Great, he's reading on grade level.  Except he's seen the movie.  In the story, the King of Thieves crashes the wedding and steals stuff, and you ask, "who is this guy?" and the kid says, "it's Aladdin's father."

 "But Aladdin doesn't know this yet, right?  He finds that out in the end.  Who does Aladdin think it is now?" 

"His father?" 

The kid can't be faulted for referencing the movie, but it never occurred to the teachers (two of them) that this was going on.

It's the same problem with the manatee story, above.  A fast reader with poor comprehension can quickly re-scan the page for the answers.  "A+!" Certainly no remedial training needed here."  But that works for a paragraph, it doesn't work for 10 pages.  I accept that he may get better, but he may not;  the point here is that a lack of comprehension goes undetected because he tests well.   By the time it is detected, it's too late.

V.

Which brings me to the real point: it would require the teachers, and the parents, to be looking for these tricks and shortcuts that kids use, and to "test" the kids in specific ways.  The immediate answer you get is, "look, in a class of 20 kids, there's just no way to test kids individually like that."  That's not the problem I'm citing here.

The problem I'm citing is that the teachers and the parents don't understand math either, because they used these shortcuts when they were kids.  I'm sure adults think they have excellent reading comprehension, but I hope a survey of the universe quickly reveals that they don't.  They get the gist.

Try this:


There are 20% more girls in the class than boys.  If there are 45 boys, how many girls are there?

Some of you will be daunted by the problem, you don't know where to start.  Interestingly, people who aren't "good at math" try and start with some abstract idea of the total.  The easier way is to start with what you know, and draw it:


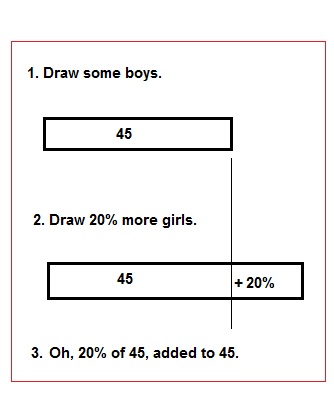
I don't know how applicable this method (Singapore math) is to students in general, but it worked for me and I'm already good at math.  And I'm sure there are other methods that are relevant to specific "hangups."  But how do you get parents and teachers to be aware of them, let alone use them?

I'm told that the system of the day is "Everyday Math." I have no idea what that is, but my worry is that every system of teaching is designed not to maximize learning, but to facilitate the teaching.  Standardize a process among teachers who themselves lack the deepest, intuitive sense of the material.  I have no idea what the solution is, though I am open to suggestions.